In: Proceedings Coastal Sediments '03. 2003. CD-ROM Published by World Scientific Publishing
Corp. and East Meets West Productions, Corpus Christi, Texas, USA. ISBN 981-238-422-7.
OTHER INLET EQUILIBRIM APPROACHES
Mota Oliveira (1970) determined from numerical experiments that for an inlet coefficient of
repletion, K, from about 0.6 to 0.8, that the bed load capacity of the tidal currents reaches a
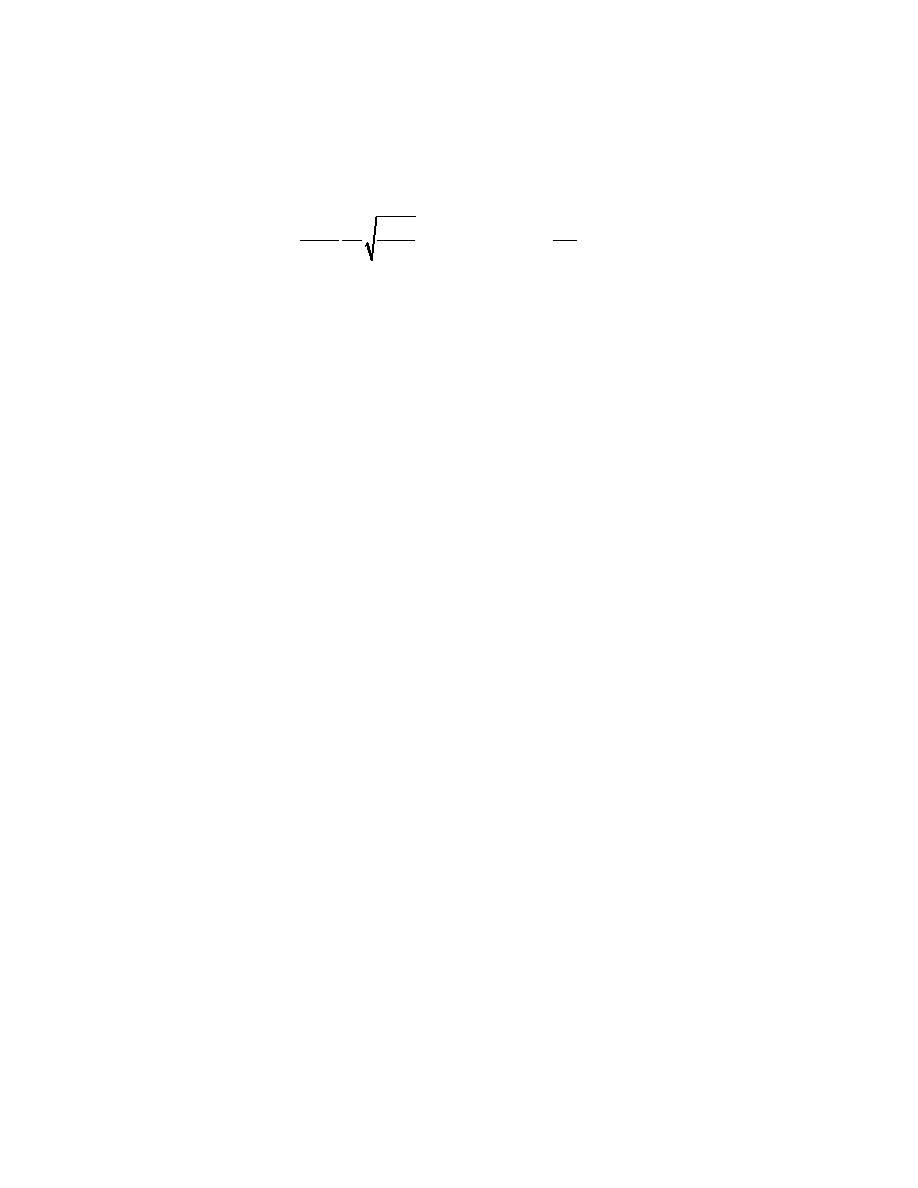
maximum. The Keulegan (1967) "K" repletion coefficient, is defined as
T Ac
2 gao
n
fL
with F = ∑ ki +
K=
(2)
2π ao Ab
F
4R
i=1
where T = tidal period; ao = tidal amplitude; Ac = the channel cross-section area; Ab = the surface
area of the bay; F= the impedance of the inlet; ki = entrance, exit and other energy loss coefficients;
f = Darcy-Weisbach friction coefficient; L = channel length; and R = hydraulic radius, usually equal
to the average depth of the channel. The repletion coefficient is roughly equal to the decimal fraction
that the bay fills, e.g., for K = 0.6, the bay fills approximately 60 %. Therefore, Mota Oliveira's
analysis supports the concept that equilibrium inlets can have bays that do not fill completely. This
phenomenon of bed load capacity efficiency can be explained by the relation of water level to the
time of maximum currents. For inlets that do not fill their bays completely, greater current
magnitudes exist for maximum ebb flow due to their occurrence at lower water levels. Flood flow is
at a higher water level, and maximum flood currents will be weaker, due to a larger channel cross-
section at the higher water level. Therefore, the seaward flushing of sediments through the inlet is
most efficient in the range of K values of about 0.6 to 0.8. This hydrodynamic process might be
expected to lead to some inlets tending to be in "equilibrium" with bays that do not fill completely.
It should be noted that other factors could contribute to whether tidal inlet channel currents are ebb
or flood dominant. For example, Boon and Byrne (1981) showed that inlets with large open bays
tend toward flood dominant currents, and inlets with bays that have highly variable areas, e.g.,
containing marsh and small channels, contribute to ebb dominant currents.
Skou (1990) examined the Escoffier curve and defined the response ability as "the most optimum
situation for an inlet to remain stable." The response ability is determined by calculating the gradient
of the Escoffier curve and plotting this slope versus the cross-section area of the inlet. The location
along the curve where the gradient was a maximum defined the area that would be able to respond to
change the fastest. Though Skou's interpretation did not define this as the equilibrium area, it was
always larger than the "critical area," i.e., the area associated with the peak of the Escoffier curve.
These two criteria will be examined for the case of a "low-K" inlet in the next section.
NON-EQUILIBRIUM INLETS (?)--AN EXAMPLE
Many inlets have low Keulegan K values. This fact indicates the inlet is not in equilibrium if the
value is below 0.6 to 0.8 (by Mota Olivera's work) and below about 2.0 (the value of K when the bay
fills completely), by Escoffier's analysis. A listing of some inlets that have or have had low K values
is shown in Table 1. When an Escoffier analysis is performed, typically the equilibrium area is
associated with the bay filling completely. An example of the Escoffier method is shown for
Barnegat Inlet, New Jersey (see Fig. 2). This inlet connects the Atlantic Ocean to a very large bay
(surface area of 123 million m2) with the entrance channel passing between two parallel jetties
spaced 305 m apart (see Figure 3). The inlet has sands ranging from 0.25 mm to 0.60 mm in
diameter. In 1968, the bay filled only 9 % of its capacity and had a Keulegan K of 0.09. Fig. 2
shows the location of the 1968 area along the stability curve. Note that it was very close to unstable
Seabergh
3
Previous Page
