solution exists (for a following current, blocking cannot occur). Inspecting
Equation 6, the right side is a linear function of the wave number (k = 2π/L),
whereas the left side is a more complicated function of k. There is only one
solution if the linear function constitutes the tangent to the function on the
left-hand side in the point of solution (Jonsson 1990), which is equivalent to
the two functions having the same gradient in k. To clarify the derivation of
the blocking condition, Equation 6 is rewritten in terms of k to yield:
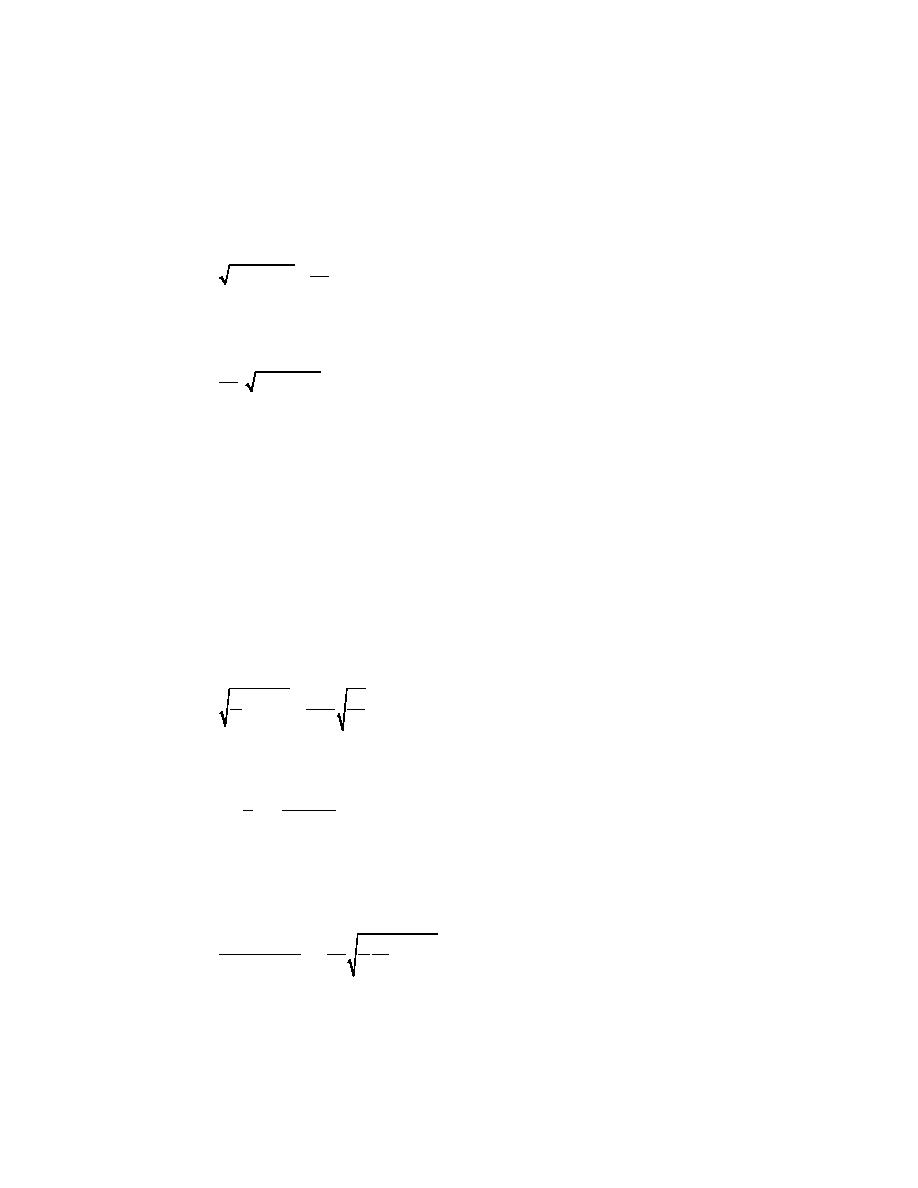
2π
gk tanh kd =
- kU cos(δ - α)
(17)
Ta
Differentiating with respect to k gives,
(
)
d
gk tanh kd = -U s cos(δ - α)
(18)
dk
where Us denotes the current speed at blocking. The left side of Equation 18
corresponds to the relative group speed, giving the following criterion for
wave blocking:
Cgr + U s cos(δ - α) = 0
(19)
This criterion implies that wave blocking occurs if the current projected on
the wave orthogonals has an opposing speed corresponding to the relative
group speed, producing an angle between the resulting direction for Cga and
the wave orthogonals of 90 deg.
may be estimated by substituting Equation 19 into Equation 6 to yield,
d
1
d
tanh kd =
(20)
1 - n Lo
L
where
1
2kd
n = 1 +
(21)
2 sinh 2kd
The required blocking speed associated with Equation 20 may be obtained
from Equation 19, once the wavelength L at blocking has been determined for
a specific Lo and d. This criterion may be written in nondimensional form as:
U s cos(δ - α)
n
Ld
=-
(22)
tanh kd
2π
gTa
h Lo
Thus, for a specific ratio d/Lo, the required blocking speed can be determined
from Equations 20 and 22. Figure 3 displays the nondimensional blocking
speed as a function of d/Lo.
18
Chapter 3 Wave Model





 Previous Page
Previous Page

