where
k = wave number (2π/L)
d = water depth (d = h+η, where h is the still-water depth
η = wave setup/setdown)
Determination of the kinematic wave properties in the presence of a
current involves solving Equations 2-5 simultaneously to obtain L, Ca, Cr,
and Tr. Thus, it is assumed that the absolute wave period is known, as well as
the current magnitude and direction (with respect to the waves, that is, δ-α).
By employing Equations 2-5, a dispersion relationship including a current
may be derived according to (Jonsson, Skovgaard, and Wang 1970),
U cos(δ - α)Ta d
d
d
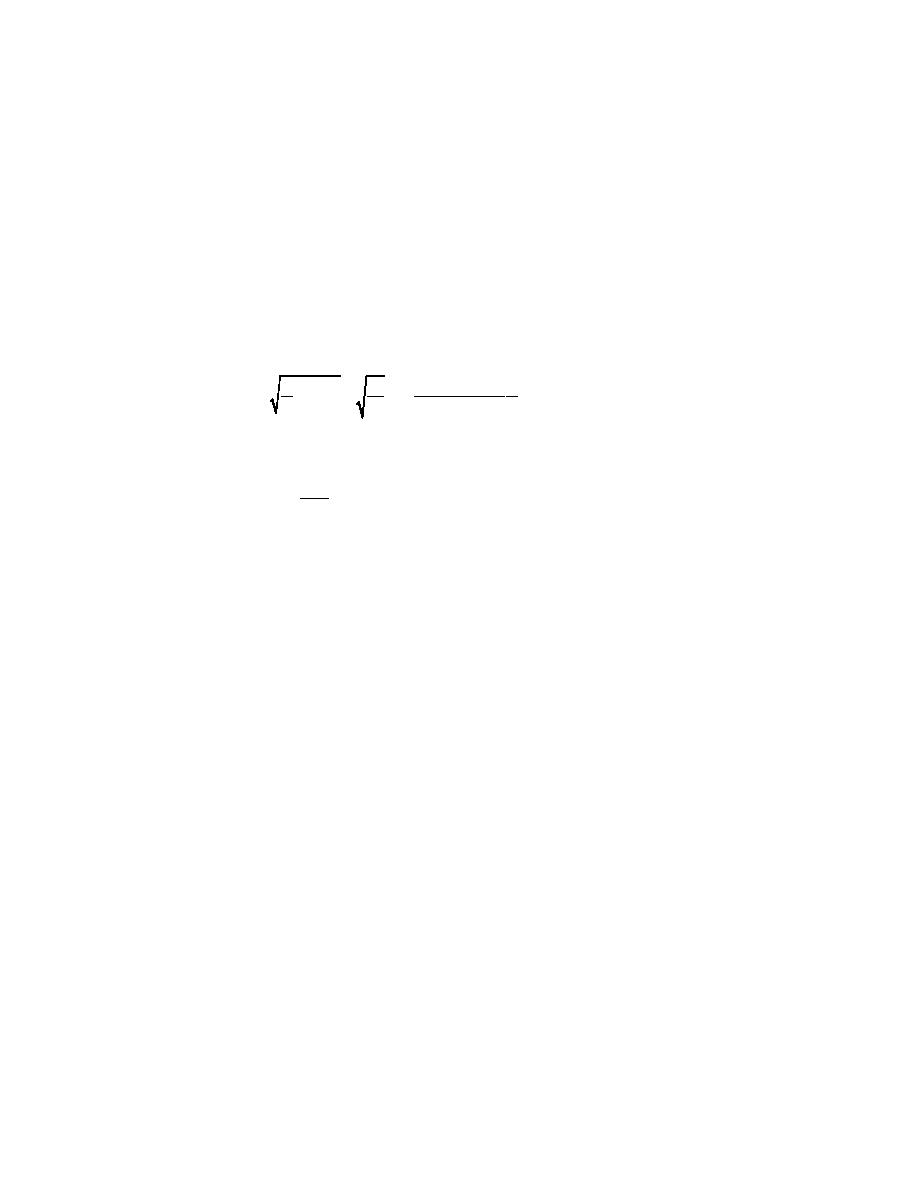
tanh kd =
1 -
L
(6)
L
Lo
d
where
gTa2
Lo =
(7)
2π
which is the deepwater wavelength for the case of no current. For a specific
absolute wave period, water depth, and current, L may be calculated from
Equation 6 through some iterative procedure. After L is known Ca is
determined from Equation 4, Cr from Equation 5, and finally Tr from
Equation 3.
Solving Equation 6 is not straightforward because the equation may have
several solutions or no solution at all, depending on the properties of the
current with respect to the wave properties. Jonsson (1990) comprehensively
discusses this topic and only a few main characteristics are pointed out here.
For the case of a following current, that is, U cos(δ-α) > 0, there are always
two solutions to Equation 6, whereas the equation can have two, one, or no
solutions for an opposing current (note that the left-hand side of Equation 6
can attain both positive and negative values for the general case).
Figure 2 illustrates typical solutions to Equation 6 for various types of
currents, plotting the right-hand side of the equation as straight lines
beginning in (d/Lo)1/2 (point A in the figure) and the left-hand side as the two
curves with decreasing gradients with increasing d/L (dashed lines in
Figure 2). For the case of waves travelling from deeper water on to a current,
it is always the solution corresponding to the lower wave number (longer
wavelength) that is physically reasonable (note that the solution
corresponding to larger d/L is not shown in Figure 2; it is located beyond the
chosen axis range). By solving Equation 6 (employing the plus sign on the
left-hand side), only the desired solution is obtained for a following current
(point B). In the situation of an opposing current, the wrong solution is
always for wave numbers larger than the wave number corresponding to
wave blocking (point E), which is discussed in the following paragraphs.
Point D indicates a solution for an opposing current not strong enough to
yield blocking. Thus, by comparing the solutions corresponding to points B,
C, and D the modification of wavelength by a current is clearly seen: a
13
Chapter 3 Wave Model





 Previous Page
Previous Page

