where the subscripts p and m represent prototype and model, respectively. The
requirement given by Equation 10 can be expressed in terms of scale ratios as:
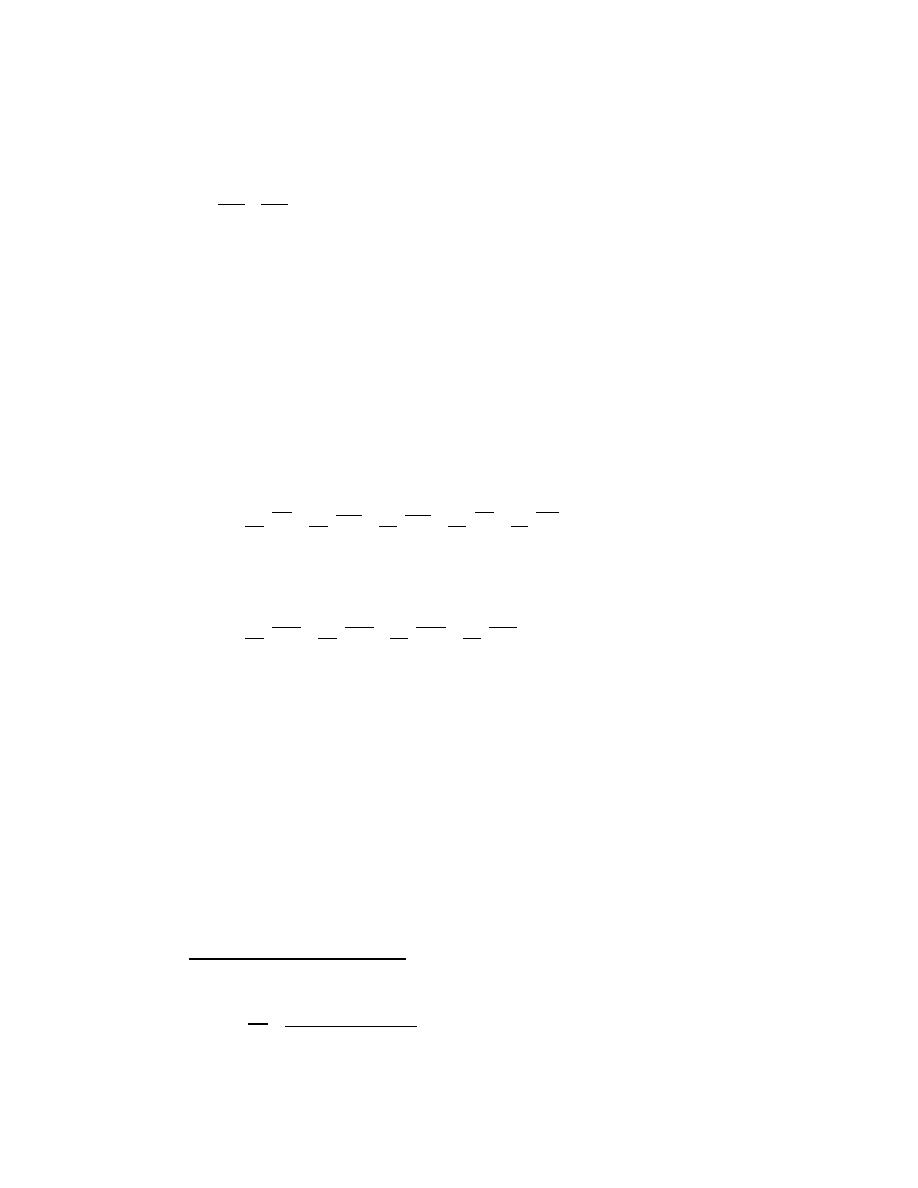
N
NX
= V
(12)
NZ NW
where, by definition, a scale ratio is the ratio of a parameter in the prototype to
the value of the same parameter in the model.
Because the scaling ratio for horizontal and vertical velocity should be the
same, the scaling requirement of Equation 4-12 can only be fulfilled when NX =
NZ, which is the requirement for a geometrically undistorted physical model.
Therefore, turbulent Reynolds stress terms containing this dimensionless
coefficient will not be in similitude in a geometrically distorted model, and this
introduces a scale effect. From Equations 4-6 through 4-9 the following can be
concluded:
turbulence terms in similitude
() ( ) ( ) () ()
∂
∂
∂
∂
∂
u '2 ;
v '2 ;
w '2
u 'v ' ;
u 'v ' ;
(13)
∂x
∂y
∂x
∂y
∂z
turbulence terms not in similitude
∂
∂
∂
∂
(
)
(
)
(
)
(
)
u ' w' ;
v 'w' ;
u 'w' ;
v 'w'
(14)
∂x
∂y
∂z
∂z
The four turbulence terms involving squares or cross products of the horizontal
turbulent velocity fluctuations and the term containing the square of the vertical
turbulent velocity fluctuation are in similitude in distorted models, whereas the
four Reynolds stress terms containing the cross-product of horizontal and vertical
velocities do not fulfill the requirement and represent the potential scale effects.
The two nonsimilar turbulent Reynolds stress terms contained in the
horizontal momentum equations are larger in the model than they should be by a
factor equal to the geometric distortion, i.e.,
1
For example, the scale ratio of the characteristic horizontal length X is:
Xp
Value of X in prototype
NX = X =
Value of X in model
m
29
Chapter 4 Turbulence Scale Effect in Distorted Models





 Previous Page
Previous Page

