and the slope dy/dx of the hyperbolic tangent for small values of x is:
dy
≈abm (b x )m- 1
(7)
dx
Therefore, the value of the slope of the hyperbolic tangent at the relative origin of
coordinates may be characterized according to the value of the coefficient m:
For m < 1, the slope is infinite (gives a symmetry point);
For m = 1, the slope is ab; and
For m > 1, the slope is zero.
Consequently, in practical application, interest lies in values of m < 1.
1000
0.0005
0.0010
0.0030
800
0.0060
600
400
200
0
0
200
400
600
800
1000
1200
1400
1600
Distance Alongshore, m
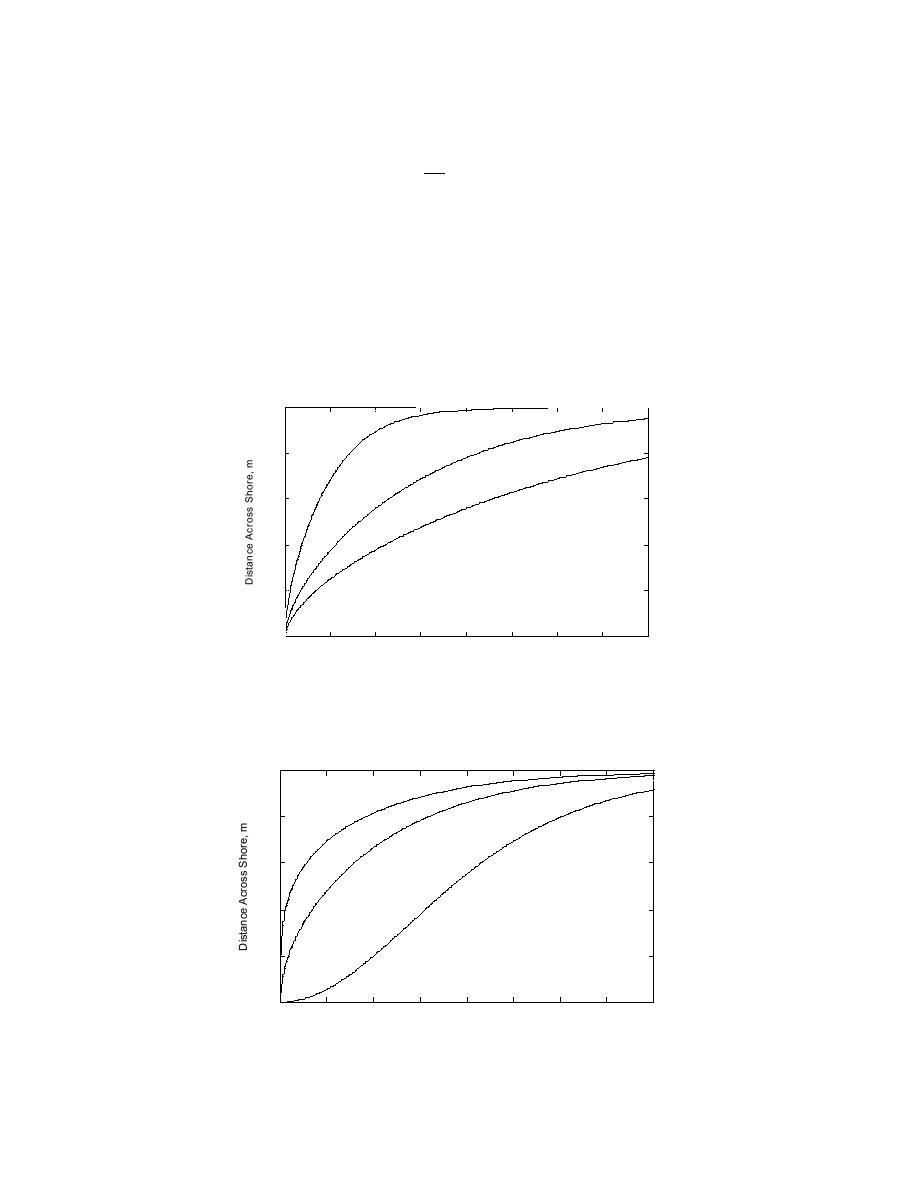
Fig. 8. Dependence of hyperbolic-tangent shape on b (a = 1,000 m; m = 0.6).
1000
0.00
800
0.25
0.50
600
400
1.00
200
2.00
0
0
200
400
600
800
1000
1200
1400
1600
Distance Alongshore, m
-1
Fig. 9. Dependence of hyperbolic-tangent shape on m (a = 1,000 m; b = 0.0012 m ).
Moreno & Kraus
10
Previous Page
