52
F.S. Buonaiuto, N.C. Kraus / Coastal Engineering 48 (2003) 5165
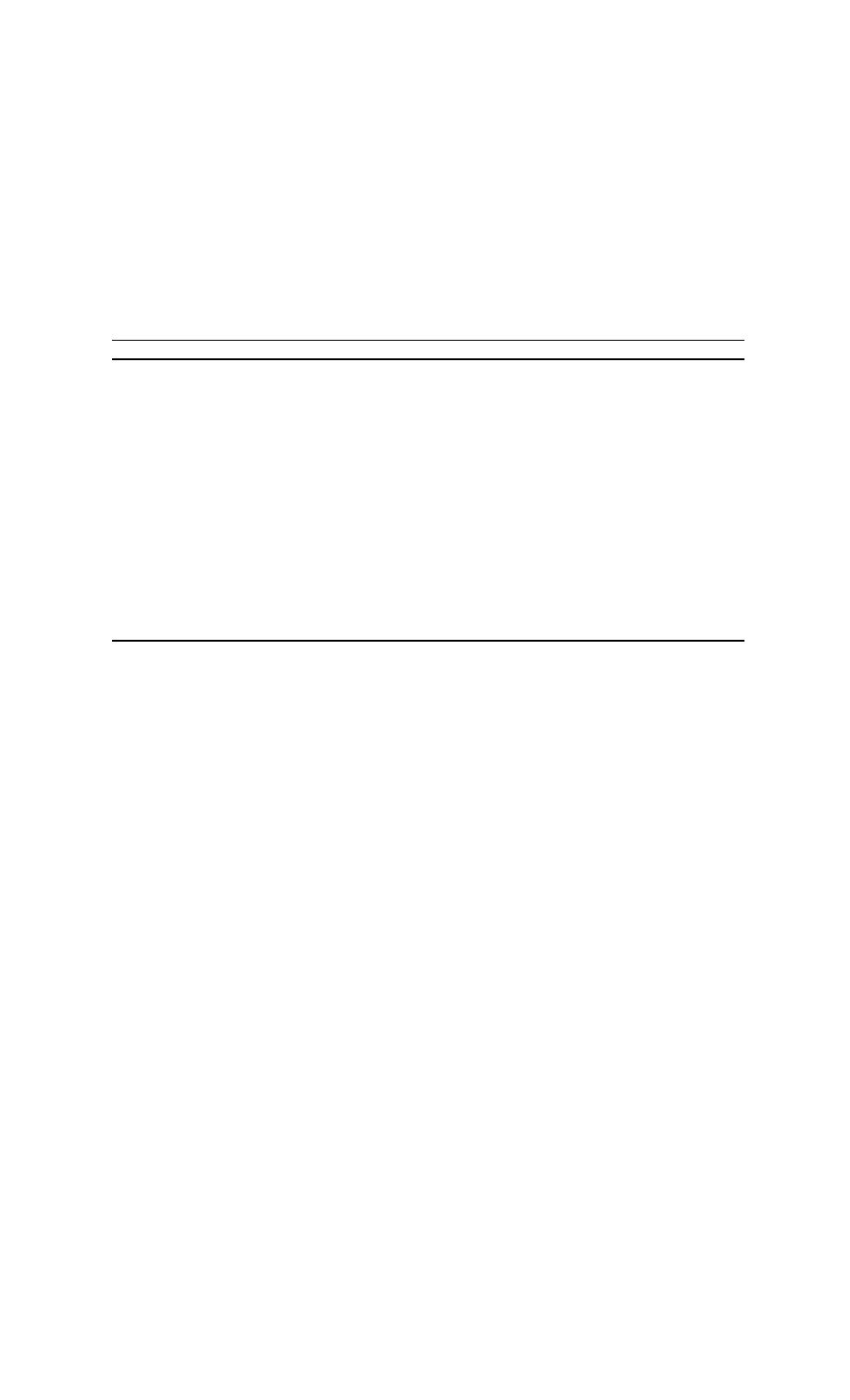
Table 1
Examples of empirical and theoretical equilibrium relationships for tidal inlet morphology
Author
Morphologic feature or relation
Relationship
AC = C1P n
LeConte (1905), O'Brien (1931, 1969),
Minimum channel cross-sectional area,
Johnson (1972); Riedel and Gourlay (1980),
AC (note: LeConte, Riedel and Gourlay,
Hume and Herdendorf (1990), etc.
and Hume and Herdendorf consider the
longshore transport rate magnitude)
Escoffier (1940)
Inlet cross-sectional area stability
Closure curve
Bruun and Gerritsen (1959, 1960)
Inlet stability, sand bypassing type
P/Qg
Floyd (1968), Floyd and Druery (1976)
Minimum entrance bar (ebb shoal)
linear
depth vs. channel depth; bar distance
offshore vs. channel depth
AC = C2P n
Jarrett (1976)
Minimum channel cross-sectional area,
with and without jetties
VE = C3P m
Walton and Adams (1976),
Equilibrium ebb shoal volume, VE (note:
Marino and Mehta (1987)
separate relations according to wave climate)
W = C4P s
Shigemura (1981)
Equilibrium throat width, W
AE = C5P k
Gibeaut and Davis (1993)
Equilibrium ebb shoal area, AE
AC = C2P n
Kraus (1998)
Derivation of minimum channel cross-sectional
area relation [note: includes longshore sediment
transport rate in C2
AF = C6P p
Carr de Betts and Mehta (2001)
Flood shoal area, AF, and volume, VF
VF = C7P q
P = tidal prism; AC minimum cross-sectional area of inlet; AE (AF) = equilibrium horizontal area of ebb (flood) shoal; VE (VF) = equilibrium
volume of ebb (flood) shoal: C = empirical or derived coefficient; k, m, n, p, q, s = empirical or derived powers; W = minimum width of inlet
throat; Qg = gross longshore transport in a year.
States, as studied here. Other relations have been
depth over the entrance bar and the depth of the
found for inlet morphology, including several describ-
entrance channel of inlets in Australia and the United
ing the tidal flats and channels of the Wadden Sea,
States. Shigemura (1981) gave a predictive relation
The Netherlands (Eysink, 1990). Summaries of the
similar to Eq. (1) for the minimum width of an
Wadden Sea empirical relations are contained in Van
unstructured (natural) inlet to the tidal prism. In a
Goor (2001) and Kragtwijk (2001).
different approach, Vincent and Corson (1981) devel-
Almost 100 years ago, Le Conte (1905) noted that
oped empirical relationships among geometric param-
the minimum cross-sectional area, AC, of an inlet
eters such as minimum depth of the ebb shoal,
channel was related to the spring tidal prism P as a
minimum inlet width, and area of the ebb delta, not
power function of the form:
considering hydrodynamic forcing.
Walton and Adams (1976) showed that the equili-
AC C1Pn
1
brium volume of the ebb shoal was also related to
tidal prism by an equation similar in form to Eq. (1),
where C1 and n are empirical coefficients, and n has a
which was further validated for inlets in Florida by
value close to unity. The form of Eq. (1) has been
Marino and Mehta (1987). Walton and Adams (1976)
verified, for example, by O'Brien (1931, 1969),
determined slightly different values of the empirical
Johnson (1972), and Jarrett (1976) for inlets in the
coefficients according to the wave energy as low,
United States; by Bruun and Gerritsen (1960), Renger
moderate, or high. Gibeaut and Davis (1993) related
and Partensky (1980), Eysink (1990), and Gerritsen et
areas of ebb shoals of selected inlets in Florida to the
al. (1990) for inlets in Europe; by Riedel and Gourlay
tidal prism. Recently, Carr de Betts and Mehta (2001)
(1980) for inlets in Australia; and by Hume and
showed that an equation of the form of Eq. (1) also
Herdendorf (1990) for inlets in New Zealand.
describes the volume of the flood shoal for selected
Floyd (1968) and Floyd and Druery (1976) found a
inlets on the east and west coasts of Florida.
linear correlation between minimum or limiting water
Previous Page
