S.A. Hughes / Coastal Engineering 51 (2004) 10671084
1073
where x is circular wave frequency (=2p/T), and T is
A convenient expression for nondimensional max-
wave period. Strictly, the dynamic pressure term
imum depth-integrated wave momentum flux arises
by dividing Eq. (11) by (qgh2) and recognizing wave
should also include a vertical velocity component
(qw2), so the dynamic pressure is at the same level
height H is twice the wave amplitude, a, i.e.,
of approximation as the horizontal velocity term.
2
MF
1 H tanhkh 1 H
However, at the wave crest, w=0 throughout the water
qgh2 max 2 h
kh
8 h
column and contributes nothing to the dynamic
!
pressure; therefore, the term is not included here.
2kh
1
12
Substituting Eqs. (8) and (9) into Eq. (7) and
sinh2kh
integrating from the bottom to the still water level
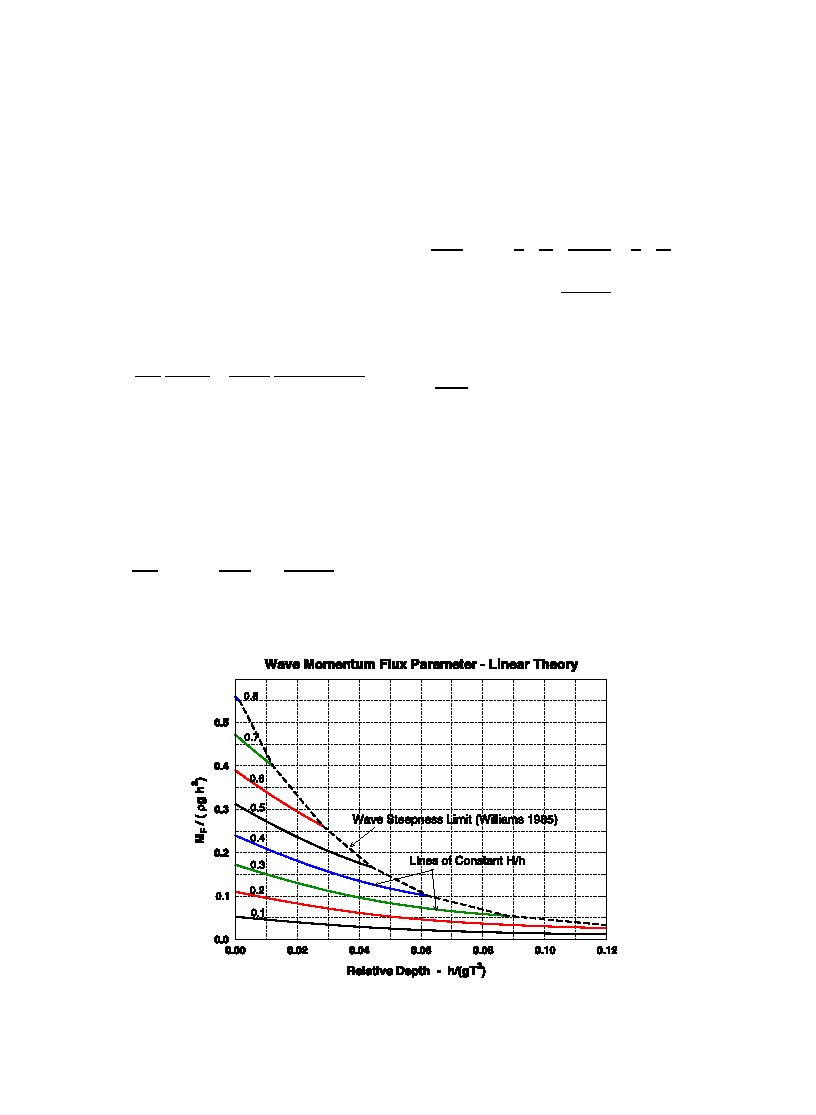
For convenience, the nondimensional depth-integrated
yields the analytically continuous expression
maximum wave momentum flux, given as
qa2x2 sinh2kh 2kh
qga sinhkh
MFmax
MF
sinh2kh
k coshkh
4k
qgh2 max
10
will be referred to as simply the bwave momentum
The second term in Eq. (10) is simplified by
flux parameter.Q
Eq. (12) expresses the wave momentum flux pa-
gk tanh kh and making use of the identity sinh
rameter as a function of relative wave height (H/h)
khcosh kh=1/2 sinh 2kh. Thus, the first-order
and relative depth (kh). Fig. 2 presents the nondimen-
theory approximation for maximum depth-integrated
sional parameter for a family of curves representing
wave momentum flux is given by
constant values of H/h. The abscissa on the plot is the
!
commonly used relative depth, h/gT2. For a constant
qga2
qga
2kh
tanhkh
MFmax
11
1
water depth, wave period increases toward the left and
k
sinh2kh
2
decreases to the right. The range of relative depths
covers most coastal applications. The dashed line
which, like Sxx, has units of force per unit length of
gives the steepness-limited wave-breaking criterion
wave crest.
Fig. 2. Wave momentum flux parameter versus h/gT2 (linear wave theory).





 Previous Page
Previous Page
