Breaking solitary wave run-up

Fig. 9. Comparison of Ahrens' (1981) and Mase's (1989) data to predictions using Egs.

Fig. 13. Measured versus predicted nonbreaking solitary wave run-up--Liformula.

Hughes-Runup-CEv51
Page Navigation
15

S.A. Hughes / Coastal Engineering 51 (2004) 10851104

1099

For a given value of the solitary wave parameter H/

h, maximum wave run-up increases for breaking

waves as the run-up slope increases. However, when

the slope becomes so steep that the waves no longer

break, further slope steepening results in decreasing

values of run-up. The transition relative wave height

between breaking and nonbreaking waves was given

by Synolakis (1986) as

0:8183cota10=9

break

Because of the difference in run-up behavior between

breaking and nonbreaking solitary waves, each case is

considered separately.

3.4.1. Breaking solitary wave run-up

Measured values of maximum solitary wave run-

up for slopes with cota=11.43, 15.0 and 30.0 were

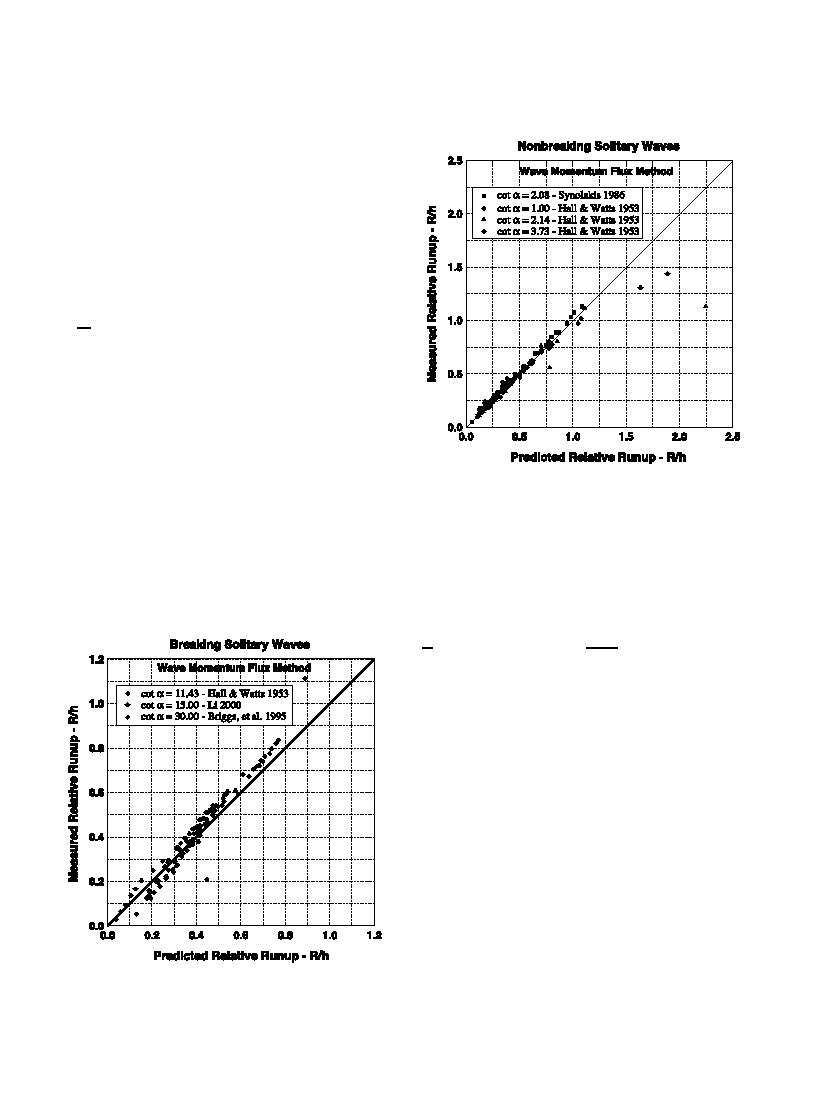
Fig. 12. Measured versus predicted nonbreaking solitary wave run-

obtained from Hall and Watts (1953), Li (2000) and

up--Eq. (44).

Briggs et al. (1995), respectively. Corresponding

values of the wave momentum flux parameter for

solitary waves were calculated for all the data. For

coefficients were then expressed as a function of slope

each structure slope an empirical coefficient was

resulting in the following simple equation for break-

determined that provided a best fit of Eq. (37). The

ing solitary wave run-up.

MF 1=2

1:39 0:027 cota

qgh2

Fig. 11 plots the 112 measured run-up values

versus run-up predicted by Eq. (43). The solid line is

the line of equivalence and the overall root-mean-

squared error was 0.051. There is reasonable corre-

spondence between estimates and observations, but

that was expected because the same data were used to

establish the predictive equation. More importantly,

there is a bias to the comparison because the simple

derivation resulted in the wave momentum flux

parameter being raised to the 1/2-power. The data

actually showed that a better fit could be obtained if

the exponent varied from 1/2 for very mild slopes up

to a value of unity near the transition between

breaking and nonbreaking waves. This implies that

the sea surface of the run-up wedge changes from a

nearly straight line to a concave shape as the structure

slope increases which agrees with run-up profiles

Fig. 11. Measured versus predicted breaking solitary wave run-up--

measured by Li and Raichlen (2001). Thus, the simple

Eq. (43).

Index

Privacy Statement
Press Release
Contact